Determining the Inertial Forces on the Camera of the SDSS Telescope

I estimated the distance between the altitude axis and the C.G. of the camera at around 37".

I was given the following numbers by Bill Boroski, FNAL:

The centrifugal force on the camera maximizes if the acceleration and velocity maximize at the bottom of the altitude axis (telescope pointing straight up). The azimuth axis does not contribute an inertial force component in this position. For constant velocity: (a = 0).

When the body is accelerating, there is an an additional term:

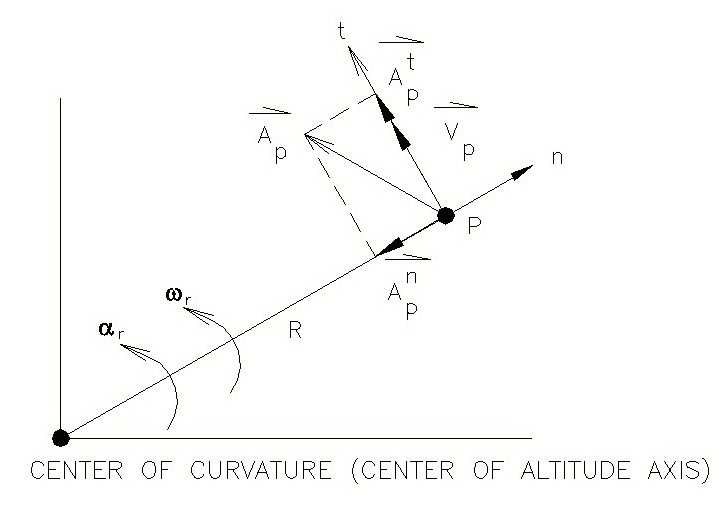
The magnitude of the first term is the previously stated rw² and it is normal to the face of the instrument rotator, so it is what the springs in the camera latches directly resist. The tangential component appears as a shear reaction in the kinematic mounts, this term does not affect the latches.

So to calculate the normal acceleration:

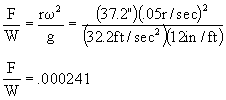
If the altitude and azimuth axes are at full speed and the altitude angle points the telescope near the horizon, then both rotational velociites would add, doubling the inertial component.

If the camera is in this configuration, its weight is a shear force on the kinematic mounts. Only at the first configuration can gravity add to the inertial component, and then the azimuthal axis component must be zero.

Conclusion:

The angular velocity of the telescope at its maximum adds a tiny component to the weight of the camera.

Last modified 04/28/99
boroski@fnal.gov